เนื้อหาของบทความนี้จะเกี่ยวกับ2 6 2 3 หากคุณกำลังเรียนรู้เกี่ยวกับ2 6 2 3มาสำรวจกันกับEOI Figueresในหัวข้อ2 6 2 3ในโพสต์Math Prof answers 6÷2(1+2) = ? once and for all ***Viral Math Problem***นี้.
Table of Contents
เนื้อหาที่มีรายละเอียดมากที่สุดเกี่ยวกับ2 6 2 3ในMath Prof answers 6÷2(1+2) = ? once and for all ***Viral Math Problem***
ที่เว็บไซต์EOI Figueresคุณสามารถเพิ่มเอกสารอื่น ๆ นอกเหนือจาก2 6 2 3สำหรับข้อมูลที่มีค่ามากขึ้นสำหรับคุณ ที่เพจeoifigueres.net เราอัปเดตข้อมูลใหม่และถูกต้องให้คุณทุกวัน, ด้วยความหวังที่จะให้บริการข่าวที่ดีที่สุดสำหรับคุณ ช่วยให้ผู้ใช้เพิ่มข้อมูลในเครือข่ายได้รวดเร็วที่สุด.
เนื้อหาบางส่วนที่เกี่ยวข้องกับหมวดหมู่2 6 2 3
ฮ่า ๆ ฉันกำลังทำสิ่งนี้จริง ๆ เหรอ? ตกลงไม่เป็นไร. มี ***ปัญหาทางคณิตศาสตร์ไวรัส*** เกี่ยวกับ เอ่อ ลำดับการดำเนินการ คุณรู้จัก #BEDMAS หรือ #PEMDAS รูปแบบที่พบมากที่สุดคือ 6/2(1+2) แต่ก็ยังแสดงเป็น 60/5(7-5) และรูปแบบอื่นที่เทียบเท่า คำตอบที่ถูกต้องที่อาจารย์คณิตศาสตร์อธิบายคืออะไร? ขอโทษ ฉันไม่สนใจ แต่ฉันยินดีที่จะแบ่งปันความคิดเล็กน้อยว่าทำไมฉันถึงคิดว่าปัญหานี้เกิดขึ้นซ้ำแล้วซ้ำเล่าซึ่งพูดถึงบางสิ่งบางอย่างเกี่ยวกับมุมมองทางสังคมเกี่ยวกับคณิตศาสตร์ เพลย์ลิสต์ VECTOR CALCULUS ของฉัน: ►VECTOR CALCULUS (Calc IV) เพลย์ลิสต์หลักสูตรอื่นๆ: ►DISCRETE MATH: ►LINEAR ALGEBRA: ►CALCULUS I: ►CALCULUS II: ►MULTIVARIABLE CALCULUS (Calc III): ►DIFFERENTIAL EQUATIONS: PLAYLISTS อื่นๆ: ► Learning Math Series ►Cool Math Series: กลายเป็นสมาชิก: ►เข้าร่วม: MATH BOOKS & MERCH I LOVE: ► My Amazon Affiliate Shop: SOCIALS: ►Twitter (อิงตามคณิตศาสตร์): ►Instagram (อิงจากการถ่ายภาพ): .
รูปภาพที่เกี่ยวข้องกับเอกสารเกี่ยวกับ2 6 2 3
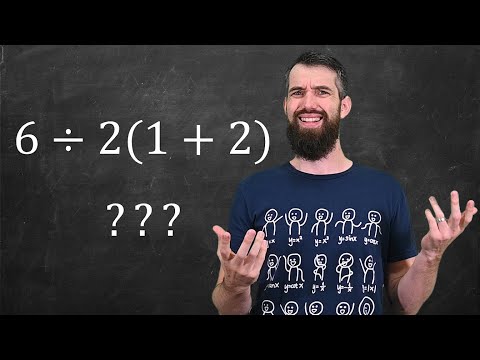
นอกจากอ่านข่าวเกี่ยวกับบทความนี้แล้ว Math Prof answers 6÷2(1+2) = ? once and for all ***Viral Math Problem*** คุณสามารถค้นพบข้อมูลเพิ่มเติมได้ที่ด้านล่าง
คลิกที่นี่เพื่อดูข้อมูลเพิ่มเติม
คีย์เวิร์ดที่เกี่ยวข้องกับ2 6 2 3
#Math #Prof #answers #Viral #Math #Problem.
Solution,Example,math,mathematics,order of operations,6÷2(1+2) = ?,The Correct Answer Explained By Math Major,math problem,division.
Math Prof answers 6÷2(1+2) = ? once and for all ***Viral Math Problem***.
2 6 2 3.
หวังว่าเนื้อหาบางส่วนที่เราให้ไว้จะเป็นประโยชน์กับคุณ ขอบคุณมากสำหรับการดูข้อมูล2 6 2 3ของเรา

Ok, you ACTUALLY want my answer? I can't just clickbait you all and not tell you which I ACTUALLY prefer? OK fine, but I can see from the comments I'm going to upset a lot of you:D If I wrote this type of thing on the board, my natural inclination is to write division as a big diagonal dash instead that lumps the 2(1+2) on the bottom. That is, when I take this algebraic string of symbols and write it out – without using any brackets – the way I would write typical calculus expressions in my classes, then I would habitually write it in a way that use spatial relationships that interpret it as being 1. If I wanted it to be 9 I'd be explicit and put brackets around the (6/2), when writing on the board. Using spatial relationships (i.e. not a strict left-to-right application of BEDMAS) is extremely common in math, it's just that normally you don't have as your starting part a character string like this because, as I say in the video, the most important part is to be explicit about what you mean when there is a possibility of ambiguity!
Ain’t no way this mf just wasted everyones time!
The answer is 1. This is not the mathematician question, is more about syntactic
"Mixed division and multiplication" – in wiki
Here is the link to it:
https://en.wikipedia.org/wiki/Order_of_operations
It’s 1 imo. The calculator literally says 1 and I got taught bidmas so brackets first.
6÷2(1+2)=9
6÷(2(1+2))=1
It's literally that simple. If you write it as a fraction with 6 over 2(1+2) you're not solving the equation as written and are instead adding that extra set of parentheses creating an ENTIRELY DIFFERENT equation resulting in 1. The correct answer is 9
This video is good, but it also misses the point of people not understanding order of operations correctly. We could clarify it, and have everyone be on the same page, but that doesn't make people actually learn the order of operations. It just makes them understand this problem and the next time they run into something slightly unclear we are back at square 0.
There is a correct way of calculating the expression as it is written, because that is what we have the order of operations for. Becasue if everyone understands the order of operations this problem is unambiguous, and clear.
And the order of operations is clear on how this is supposed to be solved. First the insides of the parentheses, and then left to right. Operation by operation, since division and multiplication has the same priority.
Making any other groupings is adding extra parentheses, and that's how simple it is.
Other mathematics professors I've seen don't avoid answering the question. While I would agree someone should avoid ambiguity, rules were made to avoid ambiguity, and for this problem ambiguity only exists for the non-cognoscenti. Ignoring the rules isn't good mathematics.
There is a right answer, that was defined early on in the 20th century.
Saying that you don't care what the answer is is avoiding the question. There is a right answer.
its obviously one
Не знаю, что он там наговорил, но ответ 1
This is obviously just a matter of convention. Once the rules are stated the ambiguity goes away, I guess everybody will agree on this. I had a professor that stated the rule pretty clear at the beginning of the course. Implicit multiplication is treated as a single number. 2(3) is the same as 2a where in this particular case a=3.
I got my master degree in 1975;that time my answer was 1 for this question. It looks like to me we are just confusing students.
Meh. If you had the personal option for rules that would be one thing. But when presented with a problem say in 6th grade and you don't interpret the problem like the teacher does, you're set up for failure. It never does any good to argue the point. And that's because it enters the 'court of opinion' which is never good especially when you want to enter a world of precision and non-ambiguity. I was always surprised that higher mathematics exists at all and the fact that YOU don't care doesn't help Me who does. This needs to be taken to a higher authority.
In math, you shouldn't ever have two 'right' answers. And you just posited you can! Nice.
Okay, so let's go to the authority on this..Excel. You'll find you can't write that expression in Excel. Well, any spreadsheet like google sheets. You would have to write it as 6/2*(1+2). And the unambiguous result is 9. Excel does not know about the properties of associated parens. This proves that you need to be literal in the expression's presentation. And that is the higher authority.
Both answers can't be correct or all of mathematics is a scam
I thought it was 1 because I thought Distributing went first because it never specifically said if distributing went first or if it acts the same as multiplication 💀
What a dork! He didn't answer.
Daniel Jung bester mann
a/b x c is not a/b x c, but a x c /b.
Pattern mdas 2 times 1= 2 is 2 2times 2 is 4 equals 6÷2+4 = next operation is division 6 divide 2 = 3+4 equals 7 7 is the answers guys dont trust my answer but its correct.
7 is the answers
yeah its 1 though
This is a great demonstration of why we never use ÷ or ⋅ when we could instead use fractions and/or parentheses
Ideal gas law PV = nRT
what’s written in textbooks or everywhere
Where n = PV/RT
Where everyone who takes physics and uses it in the future understands it stands for PV/(RT) not (PV/R)T
The bottom line is this. If you’re in a profession past primary school, the answer is 1. If you just learned pemdas in primary school and found this cool and wanted to post it online for #smartpoints, the answer is 9. Now here’s a question for you, who you gunna listen to?
When I was still a child, we were taught that multiplication came before division, so with those rules it would end up being 1.
At this precise moment I'm studying singular cohomology groups of topological spaces. That is real math, it's creating structure and finding relationships between them.
Not these stupid order-of-operation-pemdas-bombas-whattheheck problems
So if you care about effectively communicating with others, shouldn't you care that everyone looks at the math the same way since they produce different results?